6.1 Exponential functions
Exponential functions have the unknown in the exponent (power).
Exponential graphs have an asymptote at \(x=0\) and a \(y\)-intercept of \(1\).
\(y=a^x, a > 1 \)

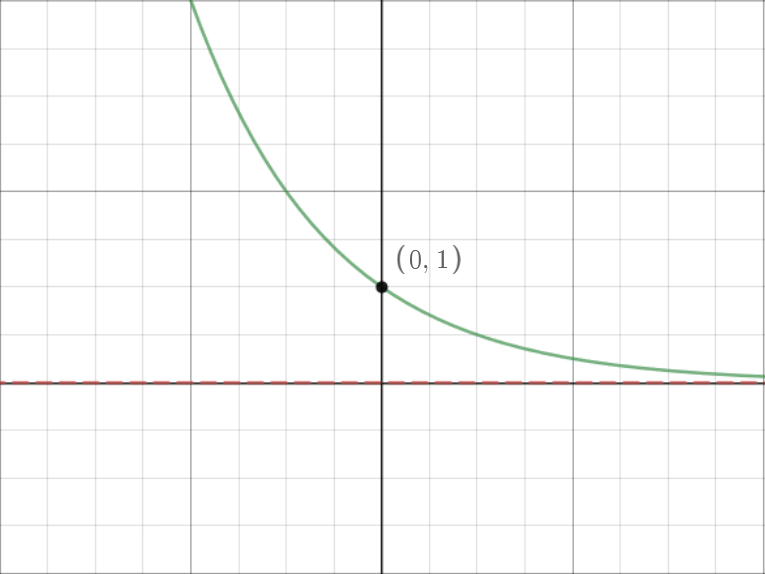
\(y=a^x, 0 < a < 1 \)
Exponential functions where \(a<0\) are beyond the scope of A-Level Maths.
The natural base
There is a special case when \(a = e\).
\(e\) is known as the natural base and is equal to \(2.71828182846...\) It is an irrational constant (similar to \(\pi\)).
\(y=e^x \)

Exponential graphs have an asymptote at \(x=0\) and a \(y\)-intercept of \(1\).
\(y=a^x, a > 1 \)

\(y=a^x, 0 < a < 1 \)

Exponential functions where \(a<0\) are beyond the scope of A-Level Maths.
The natural base
There is a special case when \(a = e\).
\(e\) is known as the natural base and is equal to \(2.71828182846...\) It is an irrational constant (similar to \(\pi\)).
\(y=e^x \)

3