5.5 Small angle approximations
Radians can be used to find approximations for the values of \(\sin{θ}\), \(\cos{θ}\) and \(\tan{θ}\).
When \(θ\) is small and measured in radians:
The graphs below show that these approximations work:
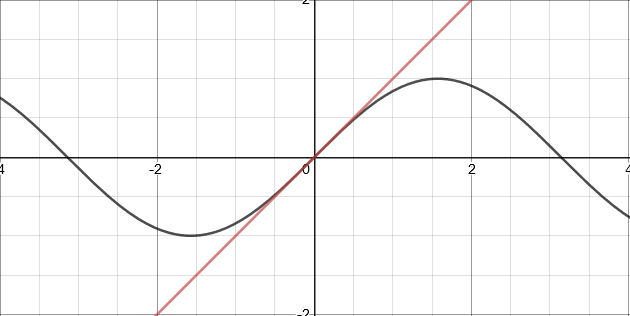
\(y=\sin{θ}\) and \(\textcolor{red}{y=θ}\)
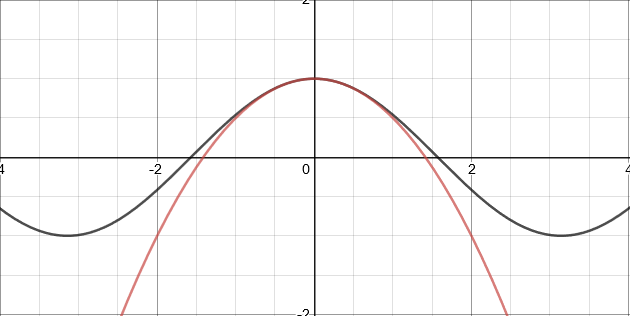
\(y=\cos{θ}\) and \(\textcolor{red}{y=1-\dfrac{θ^2}{2}}\)
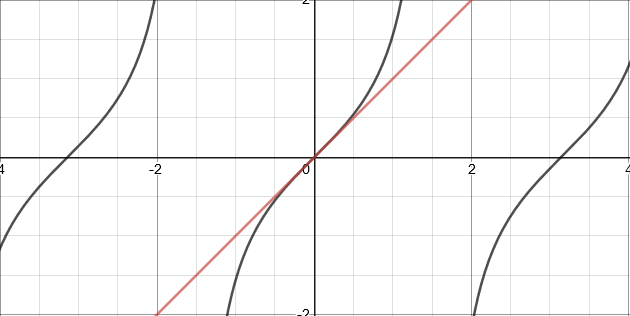
\(y=\tan{θ}\) and \(\textcolor{red}{y=θ}\)
When \(θ\) is small and measured in radians:
- \(\sin{θ} ≈ θ\)
- \(\cos{θ} ≈ 1-\dfrac{θ^2}{2}\)
- \(\tan{θ} ≈ θ\)
The graphs below show that these approximations work:
\(y=\sin{θ}\) and \(\textcolor{red}{y=θ}\)

\(y=\cos{θ}\) and \(\textcolor{red}{y=1-\dfrac{θ^2}{2}}\)

\(y=\tan{θ}\) and \(\textcolor{red}{y=θ}\)

Important
Small angle approximations
When \(θ\) is small and measured in radians:
When \(θ\) is small and measured in radians:
- \(\sin{θ} ≈ θ\)
- \(\cos{θ} ≈ 1-\dfrac{θ^2}{2}\)
- \(\tan{θ} ≈ θ\)
3